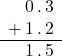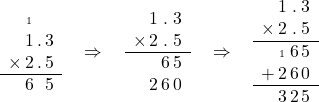MAT 111 Course Prep

Note: This page contains LaTeX markup to display mathematical notation.

This collection of problems is meant to provide practice for a range of skills needed for MAT 111. Read and attempt each problem first; if you aren’t sure how to start a problem, explore the resources on the right to refresh your memory and try again. A drop-down button is found beneath each problem for you to compare both your logic and final answers. Keep track of the skills you aren’t comfortable with, and reacquaint yourself with them so you’re fully prepared for the topics you’ll grapple with soon. Get help in the Math Lab. Return to the Course Prep page.

Simplify the following expressions:

Math Course Prep Feedback Form

Please take a few moments to fill out the anonymous feedback form below:

Problem 1	$3+7-5$ Click here to show solution. Solution: Recall the order of operations described by the acronym PEMDAS: Parentheses, Exponents, Multiplication and Division, and Addition and Subtraction. From this, we know addition and subtraction are performed simultaneously, left-to-right: $3+7-5=10-5=5.$	Video: Order of Operations Write-up: Order of Operations
Problem 2	$-(-3)-(-5)$ Click here to show solution. Solution: Distribution of both negative signs reduces this problem to $(3)+(5)$ . The answer is 8.
Problem 3	$-5(-6)$ Click here to show solution. Solution: Whenever two numbers are listed next to each other like this, it is assumed they’re being multiplied. Both factors here are negative; therefore, the final answer should be positive, and in this case it is 30.
Problem 4	$\frac{1}{2}+\frac{2}{3}$ Click here to show solution. Solution: The least common denominator (LCD) of these fractions is 6. Rewrite $\frac{1}{2}$ as $\frac{3}{6}$ and $\frac{2}{3}$ as $\frac{4}{6}$ . Then, $\frac{1}{2}+\frac{2}{3} = \frac{3}{6}+\frac{4}{6} = \frac{7}{6}$ .	Video: Finding Common Denominators Write-up: Different Denominators
Problem 5	$\frac{1}{4}\cdot \frac{5}{6}$ Click here to show solution. Solution: Multiply the numerators and denominators separately. The answer is $\frac{5}{24}$ . This fraction is in its simplest form as 5 and 24 share no common factors except 1.	Video: Multiplying Two Fractions Write-up: Multiplying Fractions
Problem 6	$0.3+1.2$ Click here to show solution. Solution: The answer is 1.5. Be sure to line up the decimal points if performing long addition: $\begin{array}{c@{\,}c@{\,}c@{\,}c}& 0 & . & 3\\+& 1 & . & 2\\\hline& 1 & . & 5\end{array}$	Video: Adding Decimals
Problem 7	$1.3\cdot 2.5$ Click here to show solution. Solution: Long multiplication gives the answer as 3.25: $\begin{array}{c@{\,}c@{\,}c@{\,}c}& \text{\tiny 1} & & \\& 1 & . & 3\\\times & 2 & . & 5\\\hline& 6 & & 5\end{array}\quad\Rightarrow\quad\begin{array}{c@{\,}c@{\,}c@{\,}c}& 1 & . & 3\\\times & 2 & . & 5\\\hline& & 6 & 5\\& 2 & 6 & 0\end{array}\quad\Rightarrow\quad\begin{array}{c@{\,}c@{\,}c@{\,}c}& 1 & . & 3\\\times & 2 & . & 5\\\hline& \text{\tiny 1} & 6 & 5\\+ & 2 & 6 & 0\\\hline& 3 & 2& 5\end{array}$ Count how many digits appear to the right of the decimal point in each term being multiplied. Here, each term has one digit to the right of the decimal point, making the total sum two digits. Therefore, the decimal point belongs in our answer where it has two digits to the right of it, meaning the answer is 3.25.	Video: Multiplying Decimals
Problem 8	$5^2$ Click here to show solution. Solution: Read as “five squared”, this can be expressed as $5*5$ . The answer is 25.
Problem 9	$16^{\frac{1}{2}}$ Click here to show solution. Solution: Raising a value to the one-half power is equivalent to taking the square root. The answer here is 4 since $4\times 4 = 16$ .	Video: Intro to Rational Exponents
Problem 10	$4x+3, \text{ given } x=2$ Click here to show solution. Solution: Plug in the value of $x$ : $4(2)+3=8+3=11$ .
Problem 11	True or False: $4 + 3 < 9 - 1$ . Click here to show solution. Solution: Simplification of each side gives $7<8$ . This is true.
Problem 12	$4+3\cdot 2-(4+7)$ Click here to show solution. Solution: Recall the order of operations discussed in Problem 1. This stipulates that expressions in parentheses are evaluated first, followed by the multiplied expression, followed by the remaining addition and subtraction. $4+32-(4+7) = 4+32-(11) = 4+6-11 = 10-11 = -1$ .	Video: Order of Operations
Problem 13	Expand: $-5(7x+4)$ Click here to show solution. Solution: Distribution (by multiplication) of the value -5 yields $(-5)(7x)+(-5)(4) = -35x-20$ .	Video: Distributive Property Write-up: Distributive Property
Problem 14	$\vert 4-9 \vert$ Click here to show solution. Solution: The subtraction yields a value of $-5$ . The absolute value, which is a strictly positive value that can be thought of as a number’s distance from zero, is simply 5 in this case.
Problem 15	$6m+8n-3m$ Click here to show solution. Solution: The commutative property of addition allows us to rewrite the problem as $6m-3m+8n = (6m-3m)+8n$ , which makes some simplification possible by combining like terms. The expression cannot be simplified beyond $3m+8n$ .